If you’re a secondary school physical science understudy, you’ve likely experienced the 5 SUVAT conditions. When acceleration is constant, these are known as kinematics equations of motion. The majority of students accept the equations without knowing where they came from and memorize them. In this article, I will demonstrate how and why I was able to derive three of the SUVAT equations using fundamental calculus.
The five SUVAT equations are as follows: s is the displacement, u is the initial velocity, v is the final velocity, a is the acceleration, and t is the time. It is important to keep in mind that the vector quantities s, u, v, and an all possess both direction and magnitude. In this unique circumstance, that implies a positive amount addresses movement in one bearing (for example upwards), and a negative amount addresses movement the other way (for example downwards).
About know more: Everything About Rzinho
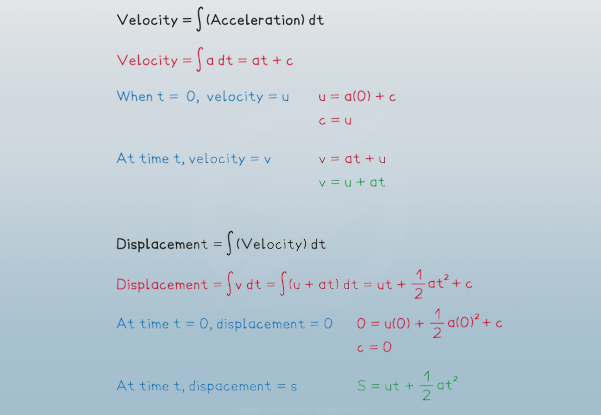
Proof 1: Since acceleration is the rate of change in velocity, v, we can say that a=dv/dt because v = u + at. DV = a dt can be obtained by multiplying both sides by dt. The result of integrating this is as follows:
At time zero, v is the same as the initial velocity, which is u. Since C = u, we can say that our equation becomes v = at + u, as required. When t = 0, at equals 0 and v = C.
Proof 2: Since velocity is the rate of change of displacement, s, we can say that s = ut + (1/2)a(t2). Ds = v dt can be obtained by multiplying both sides by dt. The result of integrating this is as follows:
Since v = u + at is what we know from equation 1, we can substitute this for:
When t is zero, s equals C. Since the object has not yet had any time to move, displacement is also zero at time zero, so C equals 0. Our condition then becomes s = ut + (1/2)a(t^2), as required.
Proof 3: We know that v2 = u2 + 2as from the first SUVAT equation. The result of rearranging and quadrupling both sides is as follows:
Do you see something recognizable? Yes! the right side of the second SUVAT equation is ut + (1/2)a(t2)! We can rewrite the initial equation as v2 = u2 + 2as, the third SUVAT equation, by using that equation to replace ut + (1/2)a(t2) with s!
I’ll let you try to figure out the last two SUVAT equations using either calculus or the other SUVAT equations now that you know how to get the first three SUVAT equations! Don’t worry if you get stuck because the fourth and fifth SUVAT equations will be proven in my next article!